Skip to main content
SpringerLink
Log in

Collocation method for solving singularly perturbed boundary-value problems by using cubic splines

  • Published:
Ukrainian Mathematical Journal Aims and scope

Abstract

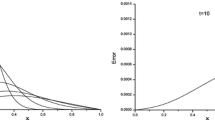
We consider singularly perturbed boundary-value problems in the case of boundary layers. To find approximate solutions of these problems, we use a collocation method based on cubic splines of minimal defect on nonuniform meshes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. G. I. Marchuk and V. I. Agoshkov,Introduction to Projective Mesh Techniques [in Russian], Nauka, Moscow (1981).

    Google Scholar 

  2. A. M. Samoilenko and N. I. Ronto,Numerical Analytic Methods for Investigation of Solutions of Boundary-Value Problems [in Russian], Naukova Dumka, Kiev (1986).

    Google Scholar 

  3. I. A. Blatov and V. V. Strygin, “Convergence of the spline collocation method on optimal meshes for singularly perturbed boundary-value problems,”Differents. Uravn.,24, No. 11, 1977–1987 (1988).

    Google Scholar 

  4. I. A. Blatov and V. V. Strygin,Convergence of the Collocation Method for Singularly Perturbed Boundary-Value Problems [in Russian], Deposited in VINITI No.4710-B87, Voronezh (1987).

  5. I. A. Blatov and V. V. Strygin, “Convergence of the spline collocation method for singularly perturbed boundary-value problems on locally uniform meshes,”Differents. Uravn.,26, No. 7, 1191–1197 (1990).

    Google Scholar 

  6. A. B. Vasil'eva and V. F. Butuzov,Asymptotic Expansion of Solutions of Singularly Perturbed Equations [in Russian], Nauka, Moscow (1973).

    Google Scholar 

  7. N. S. Bakhvalov, “On optimization of methods for solution of problems with a boundary layer,”Zh. Vych. Mat. Mat. Fiz.,9, No. 4, 841–859 (1969).

    Google Scholar 

  8. S. A. Lomov,Introduction to the General Theory of Singular Perturbations [in Russian], Nauka, Moscow (1981).

    Google Scholar 

  9. Yu. S. Zav'yalov, B. I. Kvasov, and V. L. Miroshnichenko,Spline Function Techniques [in Russian], Nauka, Moscow (1980).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Voronezh University, Voronezh

    V. V. Strygin, I. A. Blatov & I. Yu. Pokornaya

Authors
  1. V. V. Strygin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. I. A. Blatov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. Yu. Pokornaya
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Translated from Ukrainskii Matematicheskii Zhurnal, Vol. 46, No. 4, pp. 411–417, April, 1994.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Strygin, V.V., Blatov, I.A. & Pokornaya, I.Y. Collocation method for solving singularly perturbed boundary-value problems by using cubic splines. Ukr Math J 46, 433–440 (1994). https://doi.org/10.1007/BF01060413

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF01060413

Keywords

Search

Navigation