Skip to main content
SpringerLink
Log in

Simulation of spatial-temporal chaos: The simplest mathematical patterns and computer graphics

  • Published:
Ukrainian Mathematical Journal Aims and scope

Abstract

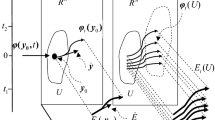
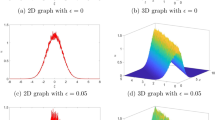
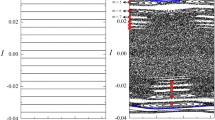
The article presents three scenarios of the evolution of spatial-temporal chaos and specifies the corresponding types of chaotic solutions to a certain nonlinear boundary-value problem for PDE. Analytic assertions are illustrated by numerical analysis and computer graphics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. H. G. Schuster,Deterministic Chaos: An Introduction, Phisik-Verlag, Weinheim (1984).

    Google Scholar 

  2. L. A. Bunimovich and A. G. Sinai, “Space-time chaos in a coupled map lattice,”Nonlinearity,1, No. 4, 491–516 (1988).

    Google Scholar 

  3. A. N. Sharkovskii, Yu. L. Maistrenko, and E. Yu. Romanenko,Difference Equations and Applications, Kluwer AP, Dordrecht (1992).

    Google Scholar 

  4. A. A. Vitt, “To the theory of a violin string,”Zh. Tekh. Fiz.,6, No. 9, 1459–1479 (1936).

    Google Scholar 

  5. A. N. Sharkovsky and E. Yu. Romanenko, “Ideal turbulence: attractors of deterministic systems may lie in the space of random fields,” in:Bifurcation and Chaos in Applied Sciences and Engineering (World Scientific),2, No. 1 (1992), pp. 31–36.

    Google Scholar 

  6. A. N. Sharkovskii, S. F. Kolyada, A. G. Sivak, and V. V. Fedorenko,Dynamics of One-Dimensional Maps [in Russian], Naukova Dumka, Kiev (1989).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Mathematics, Ukrainian Academy of Sciences, Kiev

    E. Yu. Romanenko & M. B. Vereikina

Authors
  1. E. Yu. Romanenko
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. B. Vereikina
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Published in Ukrainski Matematicheskii Zhurnal, Vol. 45, No. 10, pp. 1398–1410, October, 1993.

This investigation was partially supported by the Ukrainian State Committee for Science and Technology.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Romanenko, E.Y., Vereikina, M.B. Simulation of spatial-temporal chaos: The simplest mathematical patterns and computer graphics. Ukr Math J 45, 1569–1582 (1993). https://doi.org/10.1007/BF01571091

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF01571091

Keywords

Search

Navigation